In geometry, a polyhedron with two congruent and parallel bases is a rectangular prism. Similar to other three-dimensional shapes, a rectangular prism also has its surface area and volume.
In this article, you will find the answer to “how many edges does a rectangular prism have?” and learn more about other elements of a rectangular prism. You also learn about the important formulas of rectangular prisms.
The short answer to the above question is that a rectangular prism has 12 edges since it has 6 rectangular faces.
What is a Rectangular Prism?
A rectangular prism is a three-dimensional shape having 6 rectangle-shaped faces: one top face, one bottom face, and four lateral faces. Thus, in a rectangular prism, we have three pairs of identical faces with 6 rectangular faces, 12 edges, and 8 vertices. It also has rectangular cross-sections and equal opposite faces.
Rectangular prisms are also called cuboids because of their shape. Real-life examples of rectangular prisms include geometry boxes, notebooks, diaries, and rooms. In the below figure, we can see the shape of a rectangular prism.
Elements Of A Rectangular Prism
There are 7 elements comprising a rectangular prism, including:
- Base: There are two equal parallel bases in a prism, the top base, and the bottom base.
- Height: the perpendicular distance between the two bases.
- Axis: the line connecting the centroid of the upper and lower bases. An axis has the same length as a lateral edge.
- Lateral edge: the line created by the intersection of two lateral faces and connects the corresponding bases’ vertices.
- Lateral area: the area of the lateral faces of the prism.
- Cross section: formed by cutting the prism in a plane perpendicular to the prism’s axis.
- Vertex: refers to the point formed by three edges intersecting in a rectangular prism.
Types of Rectangular Prism
A rectangular prism can be divided into two types: right and oblique rectangular prisms.
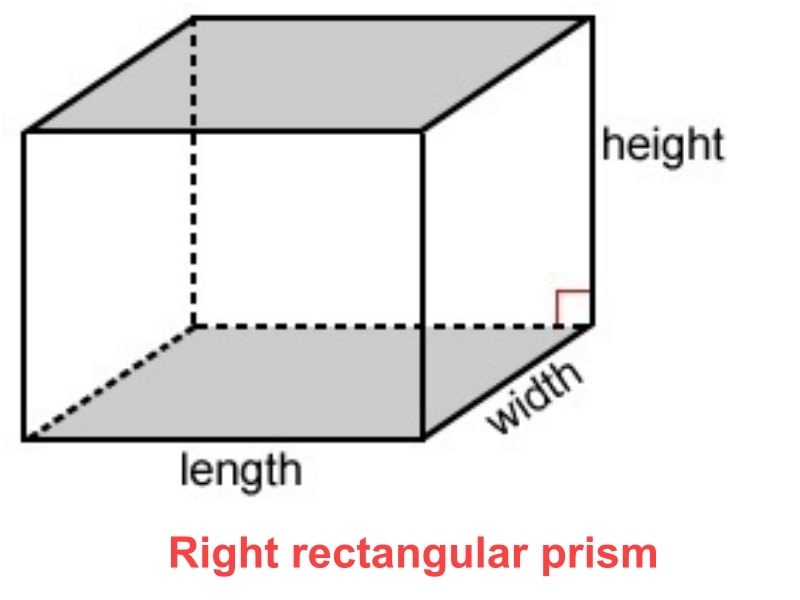
Right rectangular prism
A right rectangular prism is a prism with six rectangular faces (including two bases), and all angles are right angles, which are 90 degrees.
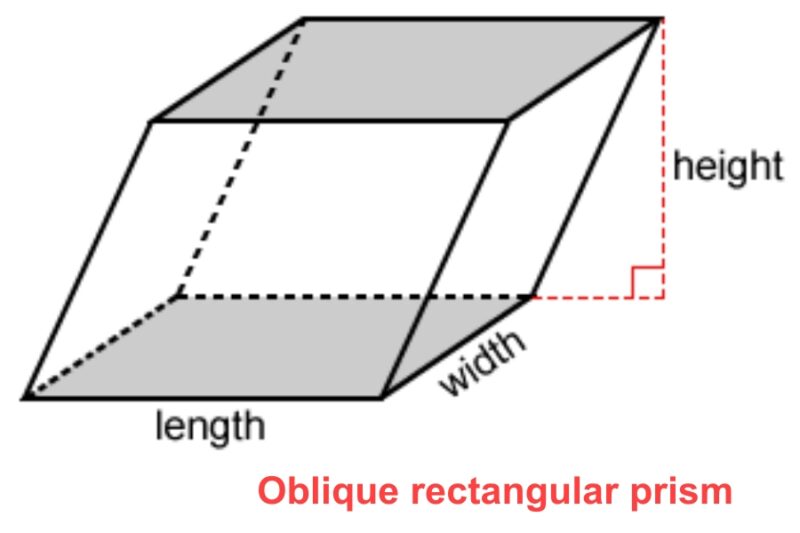
Oblique rectangular prism
The bases of an oblique prism are not perpendicular to each other and also to the lateral faces. In other words, in an oblique rectangular prism, the bases are not directly aligned.
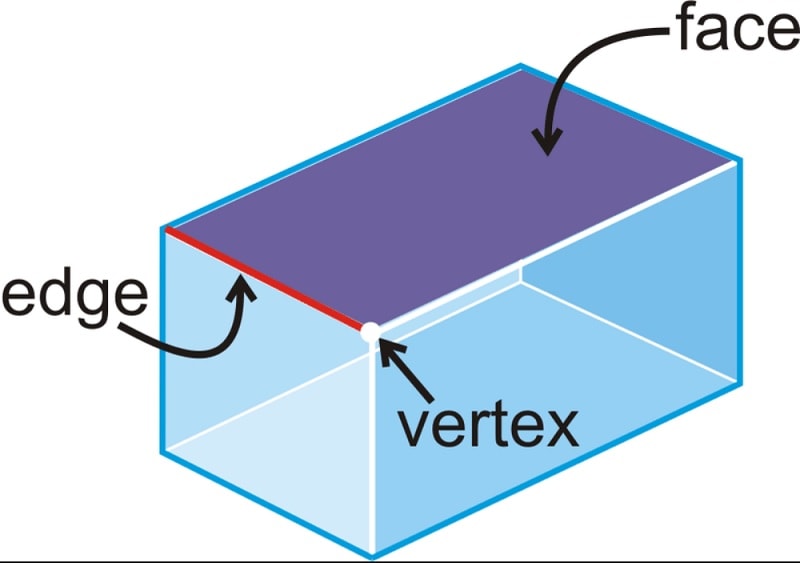
A Rectangular Prism’s Faces, Vertices, and Edges
Rectangular prism faces
A rectangular prism has six faces, all of which are planar and rectangular, meaning all the opposite faces of rectangular prisms are the same.
In right rectangular prisms, the bases meet four other lateral faces at 90-degree angles. Meanwhile, in the oblique rectangular prisms, the bases and the four lateral faces are not perpendicular.
Rectangular prism vertices
In a rectangular prism, the vertices are the points where the three edges meet. The vertices can also be defined as the points where three faces meet. So, there are 8 vertices in a rectangular prism.
Edges of rectangular prisms
Edges on a rectangular prism are lines where two faces meet. They are also the lines that connect the two vertices. So, there are 12 edges on the rectangular prism in total.
Formulas of Rectangular Prism
Volume of a rectangular prism
The rectangular prism volume is measured by cubic units. In other words, the rectangular prism volume can be defined by the total number of units used to fill a rectangular prism.
The formula to calculate the rectangular prism volume (V) is described as:
V = l x w x h
where l is the length, w is the with, and h is the height of the prism.
Surface area of a rectangular prism
The surface area of the rectangular prism surface area is defined as the sum of its faces’ area. This means the rectangular prism’s total surface area is calculated by summing the area of two rectangular bases and four lateral faces, as expressed in equation 1. The surface area is represented in square units.
Sa = LA + 2 P [1]
Let’s break it down a little bit, considering that the parallel faces in a rectangular prism have the same area.
First, we consider the lateral surface area (LA) of the rectangular prism, which is equal to the multiplication between the perimeter of the base (P) and the height (h), expressed as:
LA = P x h [2]
in which the perimeter of the base (P) can be calculated by the following formula:
P = 2 (l + w) [3]
where l represents the length of the base, and w represents the width of the base.
From expressions [2] and [3], we can draw the formula to measure the LA of the rectangular prism, as shown in the next equation:
LA = 2 (l + w) h [4]
From equations [2], [3], and [4], we can have the detailed formula for the surface area of a rectangular prism:
Sa = 2(l x w)h + 4(l + w) = 2lh + 2wh + 2 (l x h)
So, the final formula for a rectangular prism’s surface area is:
Sa = 2 (lh + wh + lw)
Net of a rectangular prism
The two-dimensional surface area of a prism when it is opened flat is its net. Imagine that we open a prism, and all its sides will be visible at once. This laid-out rectangular prism then has the net comprised of 6 rectangles, in which 2 rectangles are the bases, and the other 4 rectangles are the lateral faces, see the illustration below.
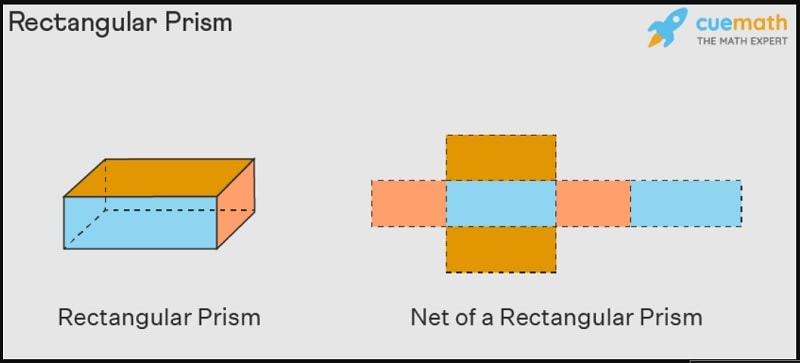
Therefore, when we use the prism’s net to calculate its surface area. The rectangular prism net (N) is computed by adding all the area of the 6 rectangles, which can be expressed as:
N = S1 + S2 + S3 + S4 +S5 + S6
in which, S indicates the area of a rectangle, which can be calculated by:
S = l x w; l is the length and w is the width of that rectangle.
Diagonal of a rectangular prism
In a rectangular prism, the diagonal runs from one corner to the opposite one. The rectangular prism diagonal (d) can be calculated using the Pythagorean theorem by knowing the prism’s length, width, and height. Therefore, we have the formula:
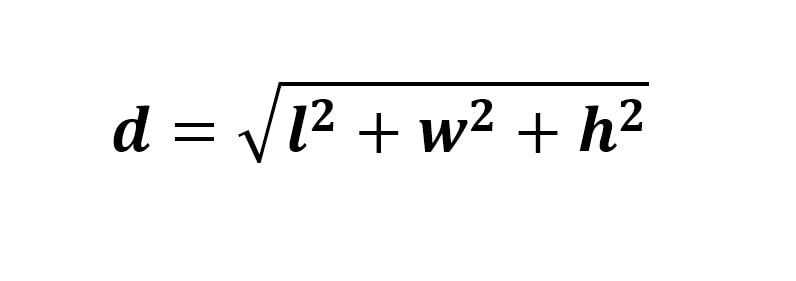
where l is the length, w is the width, and h is the length of the prism.
FAQs
Why is it called a rectangular prism?
In fact, prisms are named for the shape of their faces. So a rectangular prism is simply a prism that has rectangles as its faces. It’s an enclosed three-dimensional shape, but it’s based on two rectangles.
What objects are the right rectangular prism?
Right rectangular prisms or cuboids are all around us. A few examples are books, boxes, buildings, bricks, boards, doors, containers, cabinets, mobiles, and laptops.
Which shape is a prism?
A prism shape is a 3D shape that has a constant cross-section. Both ends have the same 2D shapes, called bases, and they’re connected by rectangular sides (except the square prism).
Video: Rectangular Prism
Conclusion
In summary, a rectangular prism is a prism with two rectangular bases. They have 6 faces in total, creating 12 edges and 8 vertices. Some examples of rectangular prisms in real life are books, boxes, buildings, and bricks. A prism can also be classified into two types: right rectangular prism, with all faces at right angles (90 degrees), and oblique rectangular prism, with two bases not perpendicular to each other.
The critical formulas for a rectangular prism are volume, diagonal, net, and surface area. Note that the length of the sides or edges needs to be given for acquiring all the rectangular prism formulas.

Tracy M. Hall was born in 1995 and studies society, human behavior, and mentality. She’s captivated by people’s interactions and motivations. After studying sociology, she got a Ph.D. in social psychology from Carnegie Mellon University. She wrote about human interaction, separation, and the future.
Tracy M. Hall is a social butterfly who likes meeting new people. She’s a superb listener and often acts as a confidante or mediator, eager to help others. Tracy’s life is an open book; Tracy shares her experiences to benefit others. She’s a natural optimist who feels everyone has something to offer and loves helping others realize their best.
Tracy M. Hall volunteered with mental health groups for years. She’s dedicated to destigmatizing mental illness and assisting.
